Sitemap

Barry's Coilguns

Mark 4

  1. Introduction
  2. Objectives
  3. Schematic
  4. Projectiles
  5. Capacitor
  6. Kinetic Energy
  7. Timing
  8. Coil Design
  9. SCR
  10. Diode
  11. Damping Resis
  12. Bleeder Resis
  13. Iron Type
  14. Iron Shape
  15. Iron Size
  16. Low Voltage
  17. High Voltage
  18. Isolation
  19. Low Voltage Power
  20. Charging Resis
  21. Construction
  22. Firing Tube
  23. Retention Bolt
  24. SCR Wiring
  25. Transformer
  26. External Iron
  27. Purchases
  28. Speed Measurement
  29. Results
  30. Coil of 97 Turns
  31. Coil of 84 Turns
  32. Damping Resistor
  33. Burned Coil #1
  34. Coil of 56 Turns
  35. Eddy Currents
  36. Starting Position
  37. Conclusions

Coil of 97 Turns

This was the first and largest of the coils tested.

Yes, I realize all these numbers and calculations are boring, but this engineering notebook includes all my raw data to permit an independent check of the calculations and conclusions later.

Coil of 97 Turns

See the Projectiles page for details of these projectiles.

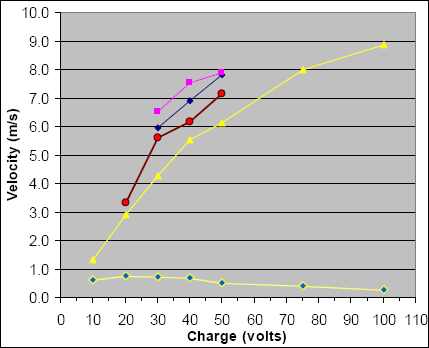
Measurements

The raw measurements are shown in red in the table below, using horizontal ballistics measurements.
Projectile:
 
A
C
D
F
Length:   3.5" 2.5" 2" 1.5"
Mass:   4.301 g 2.163 g 1.464 g 0.7345 g
Potential
energy
(joules)

Charge
(volts)
Horiz
Distance
(inches)
Horiz
Distance
(inches)
Horiz
Distance
(inches)
Horiz
Distance
(inches)
0.6 J 10 v 23" - - -
2.4 20 51 58 - -
5.4 30 75 98 104 114
9.6 40 97 108 121 132
15.0 50 107 125 137 138
33.8 75 140 - - -
60.0 100 155 - - -

Velocity

Using the equations for horizontal ballistics and the raw data, the velocity is calculated for each of the measurements above. The computed velocity is shown in red.
Projectile:
 
A
C
D
F
Potential
energy
(joules)

Charge
(volts)

Velocity
(m/s)

Velocity
(m/s)

Velocity
(m/s)

Velocity
(m/s)
0.6 J 10 v 1.316 - - -
2.4 20 2.919 3.319 - -

5.4 		30 4.292 5.609 5.952 6.524
9.6 40 5.551 6.181 6.925 7.554
15.0 50 6.124 7.154 7.840 7.898
33.8 75 8.012 - - -
60.0 100 8.871 - - -

Efficiency

Calculate efficiency as shown in red.
Projectile:
 
A
C
D
F
Potential
energy
(joules)

Charge
(volts)

Efficiency
(%)

Efficiency
(%)

Efficiency
(%)

Efficiency
(%)
0.6 J 10 v 0.6 % - - -
2.4 20 0.8 0.5 - -
5.4 30 0.7 0.6 0.5 0.3
9.6 40 0.7 0.4 0.4 0.2
15.0 50 0.5 0.4 0.3 0.2
33.8 75 0.4 - - -
60.0 100 0.3 - - -

Graphical Results

Graphical results of coil #1 with 97 turns

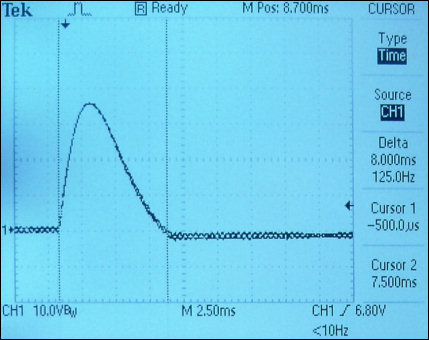
Coil Analysis

This coil with 97 turns measured 8 ms half-cycle waveform. The image below indicates peak currents of 350 A at 100v charge.

Oscilloscope image of current peak for coil with 97 turns

How Does This Coil Need to Change?

The speeds and efficiencies are very low. We need to shorten the time and increase the peak current.

From the oscilloscope, the half-cycle time is 8 ms, and we desire our next coil to be 25% faster, which means a 6 ms discharge time.

Using a Java RLC simulator, we find the estimated inductance is L = 525 uH for an 8 ms discharge with a 12,000 uF capacitor.

To reduce this to 6 ms, a Java LC Time Simulator finds that inductance should be reduced to a value of 304 uH.

We don’t want to model an iron-core coil in FEM to derive the inductance, so let’s apply the same ratio change to an air-core model. This is much easier to do, and the next bench test will tell us if this method is accurate.

  1. We need to reduce inductance L by a factor of (304 uH) / (525 uH) = 0.5790
  2. The 97-turn coil in air has inductance L = 186 uH, according to a Java Inductor Sim (using 12 AWG, ID=6mm, OD=69mm, length=16mm).
  3. The new coil in air should have L = (186 uH) * (0.5790) = 107.7 uH
  4. Using the Java Inductor Sim again, we find an 84-turn coil will have the desired inductance.
  5. Therefore, removing 13 turns should result in the desired 6 ms discharge time.
 
  < Previous Page 30 of 37 Next >